A Fuzzy Approach to the Problem of Identity

Aleksandra Kulawska – 25/09/2016

“Vagueness is endemic in the human condition” – Peter Fisher

In this essay I will argue that classical solutions to the problem of identity based on Aristotelian logic are unsuccessful because they don’t capture specific features of human reality and language. These kind of solutions are ill-fitted to solving paradoxes that arise when we think about the Ship of Theseus. In order to talk meaningfully about identity and solve the paradox, we need to focus on a fuzzier approach.

Imagine that you’re the proud owner of a beautiful ship called the Ship of Theseus. One day, you decide to remove one damaged plank from your ship and replace it with a new one. The next day, you notice another damaged plank on your ship and decide to replace it. The process continues until you decide that in order for your ship to be the best looking ship in town, you must remove all old planks and replace them with new ones. At the end of the month, you are the proud owner of a ship that’s composed of 0% of old parts, and 100% of new parts. Suddenly, a very important question arises in your mind–“is the ship in front of me the Ship of Theseus or a completely new ship?”

The identity problem (as presented above) has an identical logical structure to the sorites paradox (Douven; 2015, p. 11) and it can be mapped onto the argument structure of that paradox. Such way of presenting the sorites paradox is popular in philosophical literature (Priest 2008, Fisher 2000, Paoli 2003, Liu 2010).

Let Sx stand for the proposition: “The Ship of Theseus is the same ship after x old planks have been replaced with x new planks”. S2, for example, will then mean “the Ship of Theseus is the same ship after 2 old planks have been replaced with 2 new planks”. Let’s also assume that the Ship of Theseus is composed of 100 planks (after we remove the 100th plank, there are no original parts left in the ship).

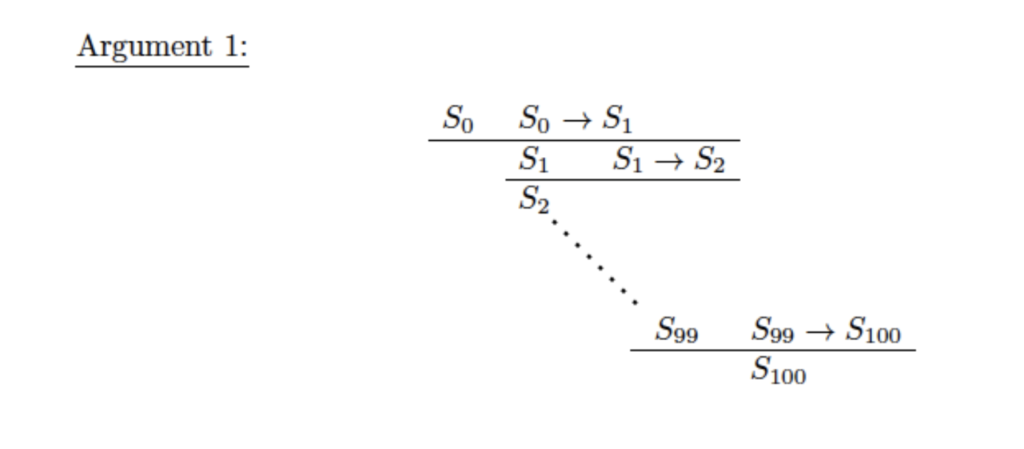
We arrive at a paradoxical conclusion–after having changed 100% of its parts, the Ship of Theseus remains the same. To put it into more perspective, let’s imagine that we were replacing old wooden planks with new, bright red plastic planks. How can a 100% wooden ship and a 100% plastic ship be identical? Also, if this is the case, how can we meaningfully talk about identity? We might think that there is something wrong with the Argument 1 – however, this is not the case. The Argument 1, as composed of modus ponens inferences, is valid. If the argument is valid, and its premises seem to be sound (1 plank doesn’t seem to make a difference), where did we go wrong?

Another issue here is that even if we could talk about the wooden ship and the plastic ship as being different, we can’t seem to be able to explain at which point the wooden ship disappears and the plastic ship comes into being. It seems impossible to point to a specific premise – after all, we agree that one plank doesn’t make a difference. It turns out that if we want to talk about the ships as being different in a meaningful way, we have to point to a very subtle difference – for example, decide that if the ship is composed of 30 plastic planks, it is the same wooden ship, but if it is composed of 31 plastic planks, it becomes the plastic ship. But, again, this seems wrong. What’s happening here?

The problem lies within the classical Aristotelian logic that we employ to think about the problem of identity and to construct the Argument 1. Aristotelian logic is based on one very important principle – the law of bivalence – which is presented below;

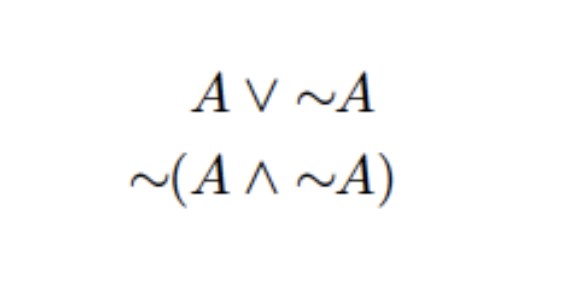
The law of bivalence states that at any given moment we can either have A, or not have A – there is no other possibility. For example, at any given moment it can either rain or not rain – we would be wrong in saying that at a given moment t, it is both raining and not raining (in the same place). This seems intuitively true. However, this sort of logic causes enormous problems when it comes

to dealing with the problems of vagueness – such as the Ship of Theseus. Let’s look again at the Argument 1. Since we only have two truth values – True and False – and cannot have anything else in between, we have to assign either T or F to each of the premises. It seems correct to say that all the premises are true – after all, it seems right to say that if the ship is the same after replacing 50 planks, it will also be the same after replacing 51 planks. This is however where we go wrong, and what leads us to a shocking conclusion. Aristotelian logic doesn’t catch the subtleties of our world and our language, which results in our having to mark each of the premises as True, which in turn leads to a valid, but intuitively false, conclusion. In order to solve the paradox, we need to employ a different kind of logic – one that would represent the subtleties and uncertainties of the world. After all, it seems that our reality isn’t black and white, comprised of either Truths or Falsities – we want to talk about things being true to a particular degree or false to some extent.

A solution to our problem is to employ one of the multi–valued logics, which give us the possibility of assigning more than one of two truth values to a given proposition. Fuzzy logic, with its degrees of truth, seems to be the most relevant in the case of identity.

What is fuzzy logic? To put it in the simplest possible way, fuzzy logic is a type of logic that allows us to assign degrees of truth to various propositions, where 1 stands for prefect truth and 0 stands for perfect falsity. For example, I can say that the proposition “Donald Trump is going to win the election” has a degree of truth 0.35. Fuzzy logic “attempts to grasp vagueness by introducing a well established and substantiated structure of truth degrees and by using the latter for modelling the way vagueness manifests itself in various situations” (Novak and Dvorak; 2011, p. 6). It also allows us to describe the world in an accurate way, as it “gives degrees of truth the same structural role as truth and falsity play in standard truth–conditional semantics” (Williamson; 2005, p. 3).

I can now show how to tackle the problem of identity using fuzzy logic. Let’s assign degrees of truth to some premises from the Argument 1.

S0: ‘The Ship of Theseus is the same ship after 0 old planks have been replaced with 0 new planks’ – this seems perfectly true; therefore, we assign the value 1 to the proposition.
S1: ‘The Ship of Theseus is the same ship after 1 old plank has been replaced with 1 new plank – since the Ship of Theseus has 100 planks, let’s say that the proposition has a degree of truth 0.99 (the ship is 99% the Ship of Theseus and 1% the new ship, at the same time). Note that this contradicts the law of bivalence – but it’s completely possible from the standpoint of fuzzy logic.
S2: degree of truth 0.98.

…

S100: ‘The Ship of Theseus is the same ship after 100 old planks have been replaced with 100 new planks’ – this seems perfectly false; therefore, the proposition has the degree of truth 0.

This seems like a very good way of talking about identity – instead of having to decide whether a thing is strictly A or not A, we can talk about it in degrees of truth. The ship with 50% new planks, is 0.50 the Ship of Theseus and 0.50 the new ship. We can think of it as being in the process of becoming the new ship, but not yet fully being the new ship. The reality of fuzzy logic is similar to that of process ontology – the world is a complicated web of processes, and instead of talking

about bivalence, we can talk about constant flux, change, becoming. No longer do we have to decide if the ship is either wooden or plastic – we can say that the wooden ship is in the process of becoming the plastic ship, with a particular degree of truth assigned to it. Using process ontology and fuzzy logic, we can meaningfully talk about identity and the persistence of things.

I can finally show how to solve the identity paradox, using a technique employed by Graham Priest to solve the original sorites paradox.

The fuzzy conditionals are usually (Priest 2000, Novak and Dvorak 2011, Chen and Pham 2001, Lee 2005) presented in the following way:

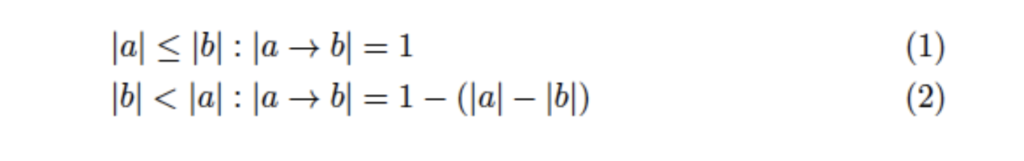
The basic idea here is that if the antecedent is less true than the consequent, the conditional is completely true – has a degree of truth 1 (Priest; 2000, p. 75). But what about validity? We have to first establish what we recognize as ‘true enough’ in a particular situation. For example, if we’re selling our ship, we want to say that we can only tell customers that it’s new if all of its planks are new (that seems fair). Therefore, our level of acceptability will be 1. However, if we’re selling our ship at a second–hand market, we might say that a ship with a value of 0.8 can be considered ‘new’. Our level of acceptability will then be 0.8. Inference is valid if the conclusion has a value as great as E (level of acceptability) in every situation, where all the premises have values at least as great as E (Priest; 2000, p. 75).

Let’s now see whether the identity paradox is valid:

In fact, all of the conditionals have degree of truth 0.99. We can now see whether the premises are true.

Let’s say that our E is 1. In order for the inference to be valid, the conclusion has to have a value at least as big as 1 and all the premises have to have values at least as big as 1. Let’s imagine that S0 has value 1 and |S0 → S1| = 1. It follows that |S1| must have value 1. In this case, the argument would be valid. However, in the paradox, each conditional has value 0.99 and S1 has value 0.99 – so the premises are unacceptable.

Let’s then say that our E is less than 1 – for example 0.8. In this case, the antecedent S0 has a value greater than E and the conditionals have value greater than E. However, the antecedent is more true than the consequent (S1 = 0.99). That means modus ponens is invalid.

In both cases, the paradox fails, which means that we no longer arrive at a valid, but paradoxical conclusion. Fuzzy logic equipped us with a meaningful way of talking about the issues of identity (degrees of truth) and also solved the paradox of identity.

It follows then, that an object that changes its parts and properties can remain the same object to a certain degree – and that, by employing fuzzy reasoning, we can talk about such object being in a process of becoming something else, and even assign a certain degree of truth to a proposition about its state.

Bibliography

Chen, G. and Pham, T. (2001) Fuzzy Sets, Fuzzy Logic and Fuzzy Control Systems. USA: CRC Press LLC.

Douven, I. (2015) ‘Verities, the sorites, and Theseus’ ship’, Synthese, doi: 10.1007/s11229-015-0724- 2.

Fisher, P. (2000) ‘Sorites paradox and vague geographies’, Fuzzy Sets and Systems, 113(1), pp. 718. doi: 10.1016/s0165-0114(99)00009-3.

Lee, K.H. (2004) First course on fuzzy theory and applications. Germany: Springer-Verlag Berlin and Heidelberg GmbH & Co. K.

Novak, V. and Dvorak, A. (2011) ‘Fuzzy Logic: A Powerful Tool for Modelling Vagueness’ University of Ostrava, Institute for Research and Applications of Fuzzy Modelling.

Paoli, F. (2003) ‘A Really Fuzzy Approach to the Sorites Paradox’, Synthese, Vol. 134, No. 3, pp. 363 387.

Priest, G. (2001) An introduction to non-classical logic. United Kingdom: Cambridge University Press.

Priest, G. (2000) Logic: A very short introduction. New York: Oxford University Press.

Williamson, T (2005) ‘Vagueness in Reality’. In Loux, M.J. and Zimmerman, D.W. (eds.) (2005) The Oxford handbook of metaphysics. New York: Oxford University Press.

Yang, L. (2010) ‘The sorites paradox and fuzzy logic’, prepared for Professor Haim Gaifman’s Seminar on Vagueness in 2010, University of Helsinki.